Here is a problem I ran across recently:
‍

Plot these numbers on the number line, and then compare them using <, >, or =‍

                                                                   40% ____ 3/10

‍

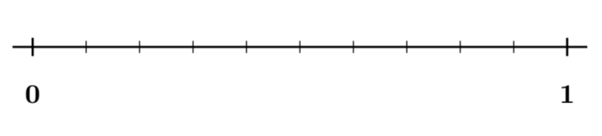
The problem seems relatively simple, and yet sent me down the rabbit hole of fractions, decimals, & percent.
‍

                          How, I wondered, can I compare them as is? 40% of what? 

‍

The number line and problem context imply 40% of 1, but is that an assumption we want our students to make?

                 And, why do I automatically ask “40% of what?”, but not “3/10 of what?”
‍

My wonderings sent me to the Common Core Standards, where I searched for “percent.” I found that in 6th grade standards, students learn to “Find a percent of a quantity as a rate per 100 (e.g., 30% of a quantity means 30/100 times the quantity); solve problems involving finding the whole, given a part and the percent.” That seems straight forward enough. Then in 7th grade, students use ratio and proportion to solve a wide variety of percent problems, but more significantly, “Students develop a unified understanding of number, recognizing fractions, decimals (that have a finite or a repeating decimal representation), and percents as different representations of rational numbers.” (https://learning.ccsso.org/wp-content/uploads/2022/11/ADA-Compliant-Math-Standards.pdf, p 46).
‍

                                               A unified understanding of number.‍

‍

A unified understanding that while 40/100 is a rational number that can be expressed as 0.4, 40% means 40 parts per 100. It is a ratio describing the relationship between a part and a whole.

The fraction 3/10, on the other hand IS a rational number, which can be written as 0.3. It has a unique address on the number line, whereas 40% 's address is dependent on the whole.

‍

What if the initial problem had looked like this?

Plot these numbers on the number line, and then compare them using <, >, or =

‍

                                                                  40%  ____  3/10

‍

‍

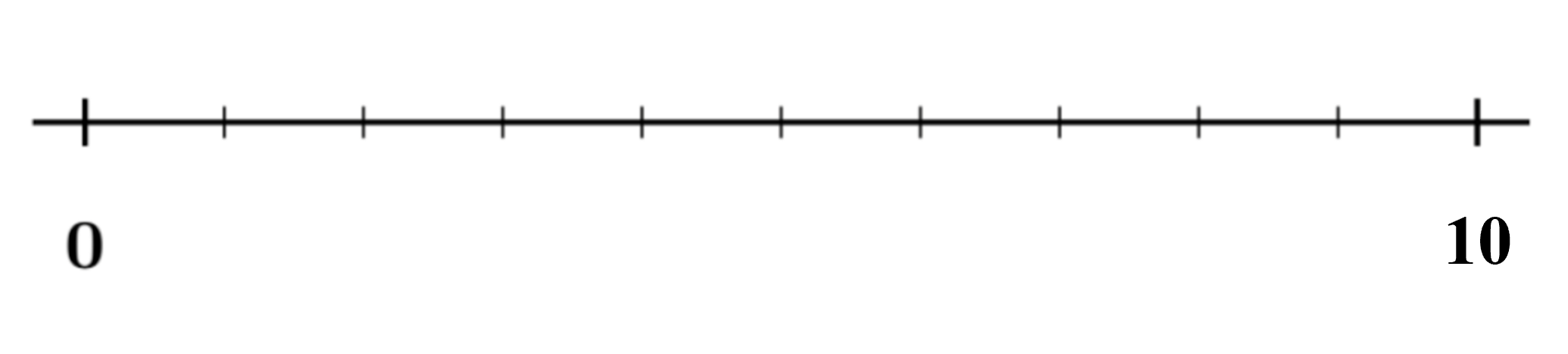
Or, what if the problem statement was:

Describe and sketch an example of:

• 40% of _____ is greater than 3/10
• 40% of _____ is equal to 3/10
• and when 40% of _____is less than 3/10

‍
‍

                                                   The crux of the matter is the whole. 
‍

The fraction 3/10 alone means 3/10 of 1, but we can also talk about 3/10 of a $24 bill or 3/10 of a 40 liter gas tank. 40% has to be 40% of something. 40% of 1, 40% of the bill, or 40% of the gas tank. 
‍

We want our students to develop deep understanding so they can flexibly navigate fractions, decimals, ratios, and percents. But how  do we help them  students develop this unified understanding of number that leads to a flexible deep conceptual understanding of rational numbers? Offer them:
‍

• Hands on experience with models such as number lines and tiles
• Problems in contexts that are culturally relevant and interesting
• Lots of time to play around and experiment as they develop their understanding.

‍

Please let me know your thoughts!

Best,
Leslie